Here’s a simple and pretty standard theory of the origin and history of life and intelligence. Life can exist in a supporting oasis (e.g., Earth’s surface) that has a volume V and metabolism M per unit volume, and which lasts for a time window W between forming and then later ending. This oasis makes discrete “advances” between levels over time, and at any one time the entire oasis is at the same level. For example, an oasis may start at the level of simple dead chemical activity, may later rise to a level that counts as “life”, then rise to a level that includes “intelligence”, and finally to a level where civilization makes a big loud noises that are visible as clearly artificial from far away in the universe.

There can be different kinds of levels, each with a different process for stepping to the next level. For example, at a “delay” level, the oasis takes a fixed time delay D to move to the next level. At a “try once” level, the oasis has a particular probability of immediately stepping to the next level, and if it fails at that it stays forever “stuck”, which is equivalent to a level with an infinite delay. And at a “try try” level, the oasis stays at a level while it searches for an “innovation” to allow it to step to the next level. This search produces a constant rate per unit time of jumping. As an oasis exists for only a limited window W, it may never reach high levels, and in fact may never get beyond its first try-try level.

If we consider a high level above many hard try-try levels, and with small enough values of V,M,W, then any one oasis may have a very small chance of “succeeding” at reaching that high level before its window ends. In this case, there is a “great filter” that stands between the initial state of the oasis and a final success state. Such a success would then only tend to happen somewhere if there are enough similar oases going through this process, to overcome these small odds at each oasis. And if we know that very few of many similar such oases actually succeed, then we know that each must face a great filter. For example, knowing that we humans now can see no big loud artificial activity for a very long distance from us tells us that planets out there face a great filter between their starting level and that big loud level.

Each try-try type level has an expected time E to step to the next level, a time that goes inversely as V*M. After all, the more volume there is of stuff that tries, and faster its local activity, the more chances it has to find an innovation. A key division between such random levels is between ones in which this expected time E is much less than, or much greater than, the oasis window W. When E << W, these jumps are fast and “easy”, and so levels change relatively steadily over time, at a rate proportional to V*M. And when E >> W, then these jumps are so “hard” that most oases never succeed at them.

Let us focus for now on oases that face a great filter, have no try-once steps, and yet succeed against the odds. There are some useful patterns to note here. First, let’s set aside S, the sum of the delays D for delay steps, and of the expected times E for easy try-try steps, for all such steps between the initial level and the success level. Such an oasis then really only has a time duration of about W-S to do all its required hard try-try steps.

The first pattern to note is that the chance that an oasis does all these hard steps within its window W is proportional to (V*M*(W-S))N, where N is the number of these hard steps needed to reach its success level. So if we are trying to predict which of many differing oases is mostly likely to succeed, this is the formula to use.

The second pattern to note is that if an oasis succeeds in doing all its required hard steps within its W-S duration, then the time durations required to do each of the hard steps are all drawn from the same (roughly exponential) distribution, regardless of the value of E for those steps! Also, the time remaining in the oasis after the success level has been reached is also drawn from this same distribution. This makes concrete predictions about the pattern of times in the historical record of a successful oasis.

Now let’s try to compare this theory to the history of life on Earth. The first known fossils of cells seems to be from 0.1-0.5 Ga (billion years) after life would be possible on Earth, which happened about 4.2 Gya (billion years ago), which was about 9.6 Ga after the universe formed. The window remaining for (eukaryotic) life to remain on Earth seems 0.8-1.5 Ga. The relatively steady growth in max brain sizes since multi-cellular life arose 0.5 Gya suggests that during this period there were many easy, but no hard, try-try steps. Multi-celluar life seems to require sufficient oxygen in the atmosphere, but the process of collecting enough oxygen seems to have started about 2.4 Gya, implying a long 1.9 Ga delay step. Prokaryotes started exchanging genes about 2.0 Gya, eukaryotes appeared about 1.7 Gya, and modern sex appeared about 1.2 Gya. These events may or may not have been the result of successful try-try steps.

Can we test this history against the predictions that try-try hard step durations, and the window time remaining, should all be drawn from the same roughly exponential distribution? Prokaryote sex, eukaryotes, and modern sex all appeared within 0.8 Ga, which seems rather close together, and leaving a long uneventful period of almost ~2 Ga before them. The clearest hard step duration candidates are before the first life, which took 0.0-0.5 Ga, and the window remaining of 0.8-1.5 Ga, which could be pretty different durations. Overall I’d say that while this data isn’t a clear refutation of the same hard step distribution hypothesis, it also isn’t that much of a confirmation.

What about the prediction that the chance of oasis success is proportional to (V*M*(W-S))N? The prediction about Earth is that it will tend to score high on this metric, as Earth is the only example of success that we know.

Let’s consider some predictions in turn, starting with metabolism M. Life of the sort that we know seems to allow only a limited range of temperatures, and near a star that requires a limited range of distances from the star, which then implies a limited range of metabolisms M. As a result of this limited range of possible M, our prediction that oases with larger M will have higher chances of success doesn’t have much room to show itself. But for what its worth, Earth seems to be nearer to the inner than outer edge of the Sun’s allowable zone, giving it a higher value of M. So that’s a weak confirmation of the theory, though it would be stronger if the allowed zone range were larger than most authors now estimate.

What about volume V? The radii of non-gas-giant planets seems to be lognormally distributed, with Earth at the low end of the distribution (at a value of 1 on this axis):

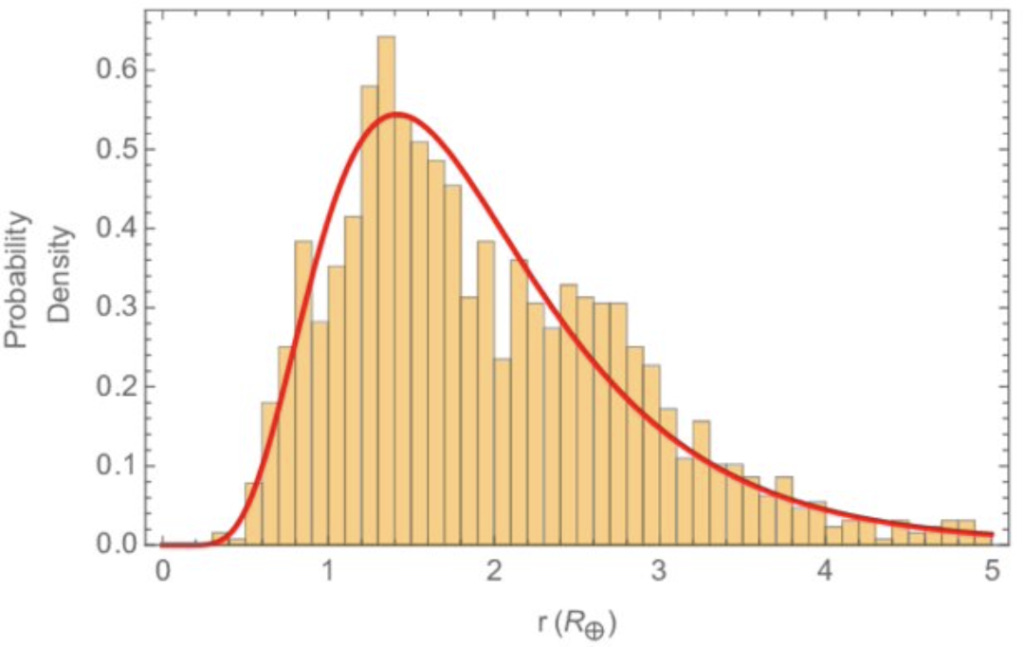
So there are many planets out there (at r=4) with 16 times Earth’s surface area, and with 64 times the volume, ratios that must be raised to the power of N to give their advantage over Earth. And these larger planets are made much more of water than is Earth. This seems to be a substantial, if perhaps not overwhelming, disconfirmation of the prediction that Earth would score high on VN. The higher is the number of hard steps N, the stronger is this disconfirmation.

Regarding the time window W, I see three relevant parameters: when a planet’s star formed, how long that star lasts, and how often there are supernova nearby that destroy all life on the planet. Regarding star lifetimes, main sequence star luminosity goes as mass to the ~3.5-4.0 power, which implies that star lifetimes go inversely as mass to the ~2.5-3.0 power. And as the smallest viable stars have 0.08 of our sun’s mass, that implies that there are stars with ~500-2000 times the Sun’s lifetime, an advantage that must again be raised to the power N. And there are actually a lot more such stars, 10-100 times more than of the Sun’s size:

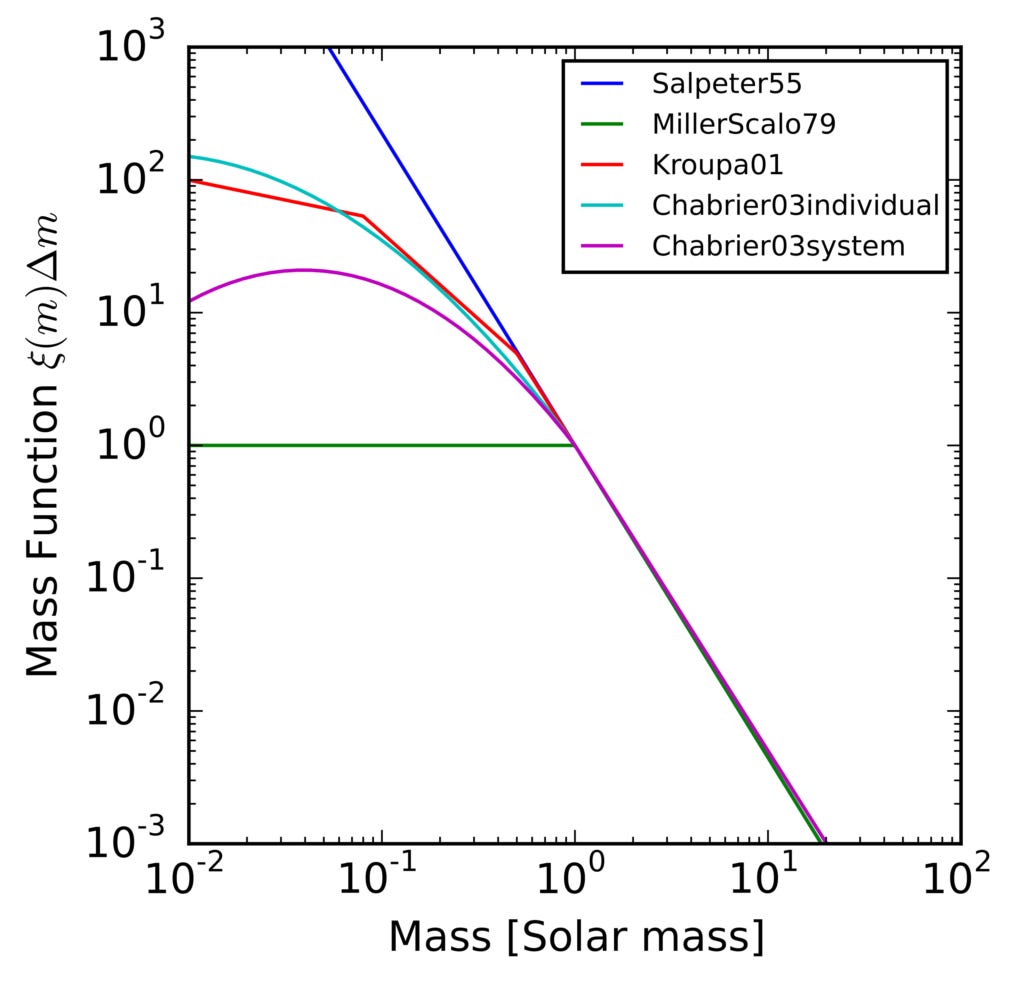
However, the higher metabolism of larger mass stars gives them a spatially wider habitable zone for planets nearby, and planets near small stars are said to face other problems; how much does that compensate? And double stars should also offer wider habitable zones; so why is our Sun single?

Now what if life that appears near small long-lived stars would appear too late, as life that appeared earlier would spread and take over? In this case, we are talking about a race to see which oases can achieve intelligence or big loud civilizations before others. In which case, the prediction is that winning oases are the ones that appeared first in time, as well has having good metrics of V,M,W.

Regarding that, here are estimates of where the habitable stars appear in time and galactic radii, taking into account both star formation rates and local supernovae rates (with the Sun’s position shown via a yellow star):

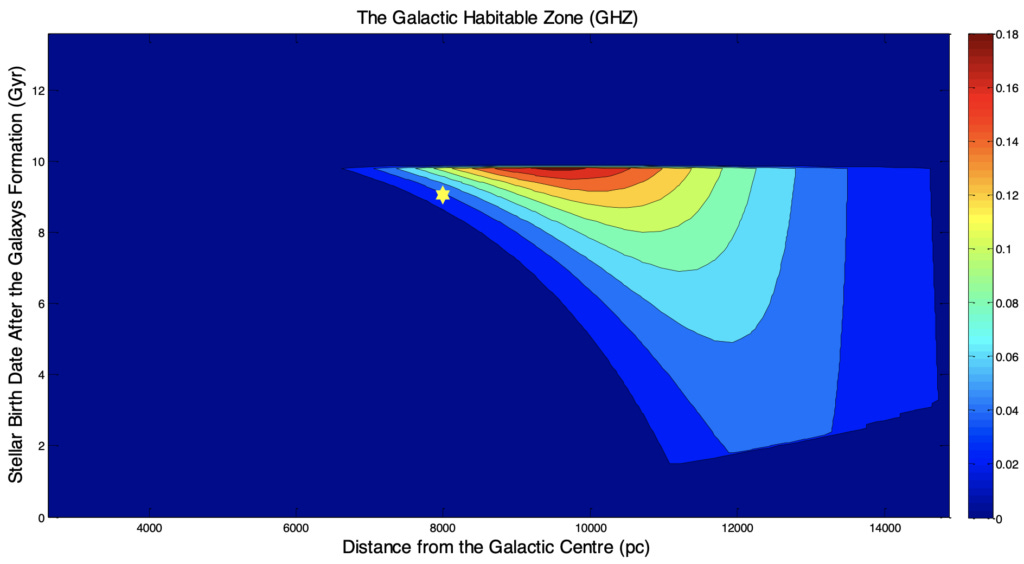
As you can see, our Sun is far from the earliest, and its quite a bit closer to galactic center than is ideal for its time. And if the game isn’t a race to be first, our Sun seems much earlier than is ideal (these estimates are arbitrarily stopped at 10Ga).

Taken together, all this seems to me to give a substantial disconfirmation of the theory that chance of oasis success is proportional to (V*M*(W-S))N, a disconfirmation that gets stronger the larger is N. So depending on N, maybe not an overwhelming disconfirmation, but at least substantial and worrisome. Yes, we might yet discover more constraints on habitability to explain all these, but until we find them, we must worry about the implications of our analysis of the situation as we best understand it.

So what alternative theories do we have to consider? In this post, I’d like to suggest replacing try-try steps with try-once steps in the great filter. These might, for example, be due to evolution’s choices of key standards, such as the genetic code, choices that tend to lock in and get entrenched, preventing competing standards from being tried. The overall chance of success with try-once steps goes as the number of oases, and is independent of oasis lifetime, volume, or metabolism, favoring many small oases relative to a few big ones. With more try-once steps, we need fewer try-try steps in the great filter, and thus N gets slower, weakening our prediction conflicts. In addition, many try-once steps could unproblematically happen close to each other in time.

This seems attractive to me because I estimate there to be in fact a great many rather hard steps. Say at least ten. This is because the design of even “simple” single cell organisms seems to me amazingly complex and well-integrated. (Just look at it.) “Recent” life innovations like eukaryotes, different kinds of sex, and multicellular organisms do involved substantial complexity, but the total complexity of life seems to me far larger than these. And while incremental evolution is capable of generating a lot of complexity and integration, I expect that what we see in even the simplest cells must have involved a lot of hard steps, of either the try-once or the try-try type. And if they are all try-try steps, that makes for a huge N, which makes the prediction conflicts above very difficult to overcome.

Well that’s enough for this post, but I expect to have more to say on the subject soon.

Added 19Jan: Turns out we also seem to be in the wrong kind of galaxy; each giant elliptical with a low star formation rate hosts 100-10K times more habitable Earth-like planets, and a million times as many habitable gas giants, than does our Milky Way.