The simulation argument was famously elaborated by Nick Bostrom. The idea is that our descendants may be able to create simulated creatures like you, and put them in simulated environments that look like the one you now find yourself in. If so, you can’t be sure that you are not now one of these future simulated people. The chance that you should assign to this possibility depends on the number of such future creatures, relative to the number of real creatures like you today.
More precisely, let P be the fraction of descendant civs that become able to create these ancestors simulations, I the fraction of these that actually do so, N the average number of ancestors simulated by each such civ per ancestor who once existed, and S the chance that you are now such an ancestor sim. Bostrom says that S = P*I*N/(P*I*N+1), and that N is very large, which implies that either P or I is very small, or that S is near 1. That is, if the future will simulate many ancestors, then you are one.
However, I will now show that this argument collapses if we allow the inclination to simulate ancestors to depend on the time duration that has elapsed between those ancestors and the descendants who might simulate them. My main claim is that our interest in the past generally seems to fall away with time faster than the rate at which the population grows with time. For example, while over the last century world population has doubled roughly every 40 to 60 years, this graph shows much faster declines in how often books mention of each of these specific past years: 1880, 1900, 1920, 1940, 1960.
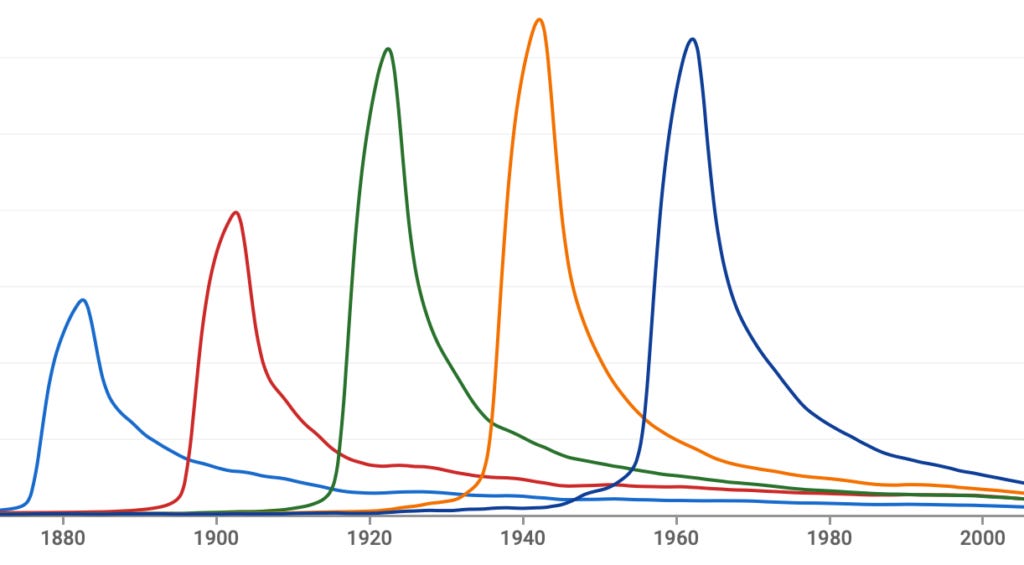
Let us now include this fading past effect in a simple formal model. Let t denote a cultural “time” (not necessarily clock time), relative to which population (really a density of observer-moments) grows exponentially forever, while interest in the past declines exponentially. More formally, assume that it is already possible to create ancestor sims, that population grows as eg*t, that a constant fraction a of this population is turned into simulated ancestors, and that the relative fraction of these simulated ancestors associated with simulating a time t units into the past goes as e–b*g*t. Thus for b>1 per-person interest in past people falls as e-(b-1)*g*t.
Given these assumptions, the ratio of future ancestors simulations of the current population to that actual current population is F = a*b/(b-1), and S = F/(F+1). So, for example, if at any one time 10% of people are ancestor simulations, and if interest in the past falls by 12% every time population rises by 10%, then a = 0.1, b = 1.2, and F = 0.6, giving each person who seems to be real a S = 3/8 chance of instead being an ancestor simulation. If a = 0.001 instead, then F = 0.006, and each person should estimate a S =~0.6% chance of being an ancestor simulation.
The above assumed that ancestor sims are possible and are being done now. If instead sims can’t start being created until c time units in the future, then we instead have F = a*(b/(b-1))*e(b-1)*g*c, giving an even smaller chance S of your being an ancestor simulation.
By the way, these calculations can also be done in terms of rank. If all people in history are ordered in time, with r=0 being the first person ever, and all others having r>0, then we could assume that a fraction a of people are always ancestor simulations, and that interest in past people falls as rb, and we’d again get the same result F = a*b/(b-1).
Thus given the realistic tendency to have less interest in past people the further away they are in time, and the likely small fraction of future economies that could plausibly be devoted to simulating ancestors, I feel comfortable telling you: you are most likely not an ancestor simulation.
Added 9pm: See this more careful analysis by Anders Sandberg of falling interest in year names. Seems to me that fall in interest is in fact faster than the population growth rate, even a century after the date.



Interesting argument. More generally I believe that this kind of reasoning on probability distributions of simulations can put strong constraints on the simulation hyphotesis.
My problem with it is that it's ignoring other factors which make simulations more likely to be run (a.k.a Simplicity Assumption), in particular the computational complexity of the simulations. If a simulation is 1000x easier to simulate, just few simulators are enough to quickly make easier simulations dominant. Imagining that nobody in the future will take a shot at simulating very easy sims is unlikely, similarly to how Fermi's paradox cannot be solved with "ALL possible aliens will do X".
For more info on this line of reasoning check https://philpapers.org/rec/...
My math above does integrate over the entire future.