I recently came across this news item:
Factoring in gravitomagnetism could do away with dark matter
By disregarding general relativistic corrections to Newtonian gravity arising from mass currents, … Ludwig asserts [standard] models also miss significant modifications to [galaxy] rotational curves … because of an effect in general relativity not present in Newton’s theory of gravity — frame-dragging … Ludwig presents a new model for the rotational curves of galaxies which is in agreement with previous efforts involving general relativity. … even though the effects of gravitomagnetic fields are weak, factoring them into models alleviates the difference between theories of gravity and observed rotational curves — eliminating the need for dark matter.
My initial reaction was skeptical snark. Yes, gravity has a magnetism, just as does electricity, and yes magnetism can push on stuff in a way that mimics the effects of dark matter. But I knew that this is an old hope, usually dropped after people do a standard quick calculation and see that its effect looks really weak, given the usual speeds of stars rotating in a galaxy.
But then a few days later I actually read the paper, and found myself impressed and persuaded. When I tweeted this fact, I got a lot of indignant pushback. Many said there’s no point to a paper that explains galaxy rotation curves, if it doesn’t also explain all the other data said to support dark matter. Many publicly said that the paper is almost surely wrong, because of the usual quick calculation. For example Garrett Lisi posted this:

Yet I could prod few of these denouncers to actually read the paper. (And most who did seemed to fail some basic comprehension tests.) Some even said I have too few physics journal publications (only 3) to speak publicly on the topic; I should leave that to those who refused to read the paper.
But the whole point of news and research is be surprised to learn things you’d didn’t expect. Why even have news or research if you will only allow them to confirm what you expect? The author, Gerson Ludwig, is well aware of the usual expectations, and published his finding saying they are wrong in a good peer reviewed journal. Furthermore, Ludwig is part of a research tradition of a least 5 papers I’ve found (1 2 3 4 5), all of which say there’s much less need to invoke dark matter to explain galaxy rotation curves if one does calculations closer to full general relativity. If even after that everyone is going to reject the idea based on priors and a quick heuristic calculation, why do research?
So I decided to dig into this paper, to see if I couldn’t either find its mistake or explain its reasoning better. Bottom line: I found a big very questionable assumption made not only by Ludwig, by also by the other 4 papers. See if you can spot it before I tell you.
For planets orbiting stars, or moons orbiting planet, it is widely accepted that simple Newtonian gravity is an excellent approximation. But when this approach was used to study orbits of stars around galaxies, it was found to badly predict their orbital speeds (i.e., “rotation curves”). To explain this puzzle, many posit a lot more “dark matter” than what is easily seen, distributed quite differently than the stuff we easily see.
Even though the usual quick calculation suggests it won’t make a difference, a number of authors have tried to calculate these rotation curves using something closer to (but still far from) full general relativity (GR). And all of those (that I’ve found) claim that it makes a big difference, enough to solve the puzzle. For which they are also widely criticized, because priors and usual quick calculation.
Ludwig tried a standard approximation to GR that is closer than Newton, but still linear, one which we understand well as it is very like Maxwell’s equations for electromagnetism:
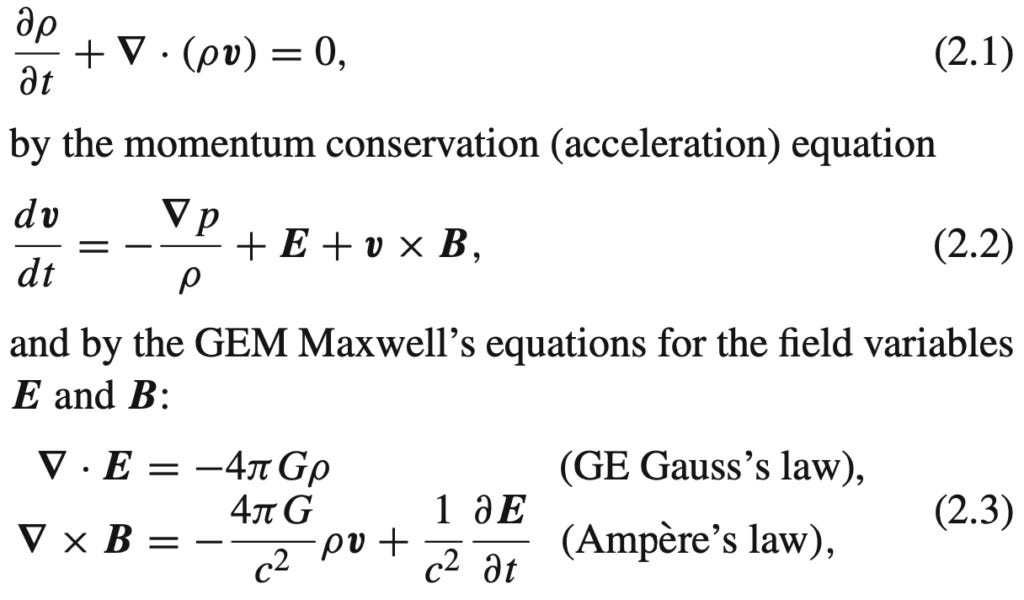
Here E is gravity’s “electric” field that pushes still stuff toward each other, and B is gravity’s “magnetic” field, that in addition pushes away from each stuff that is moving in parallel.
As star-star collisions are very rare, Ludwig assumes a time-invariant (i.e., “equilibrium”) rotationally-symmetric system of zero-pressure (p=0) dust that only moves in the azimuthal direction. (That is “around” the galaxy, in a direction perpendicular to the radial and vertical dimensions). This implies that E and B have only radial components ER, BR and vertical components EZ, BZ, and also that:

The first (radial balance) equation says that magnetism is only a big effect on star motions in galaxies if vB becomes comparable in magnitude to E, while the second (axial balance) equation says that E and vB are in fact comparable in magnitude! Yes these are talking about different (R vs. Z) components of these vectors, but over the whole galaxy these components are connected in ways that ensure that large values of one component in one place imply large values of the other component in other related places. For example, here is a calculated B field around a spinning uniform mass sphere:
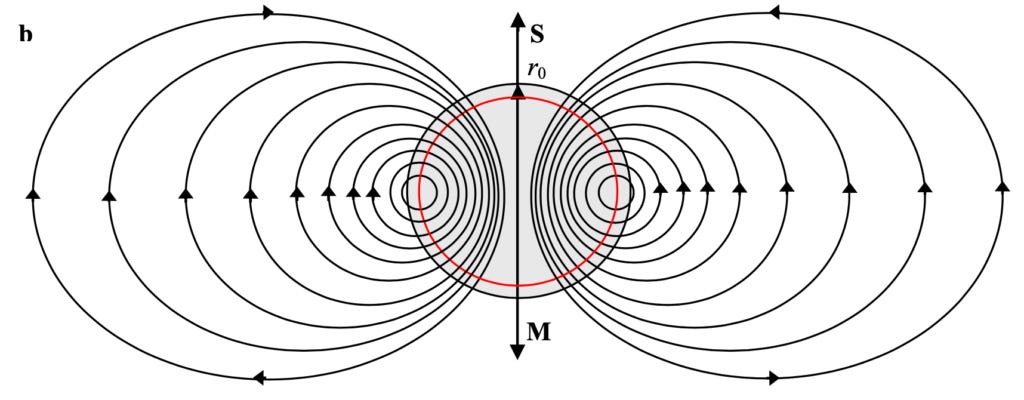
Thus Ludwig finds gravitomagnetism to be always important for equilibrium rotating gravitating dust! Using his model, he does a decent job of predicting rotation curves for three galaxies, using only mass distributions estimated from the light we see, though he allows some corrections and fits the ratio of mass to light to each galaxy.
So how could the usual quick calculation go so wrong here? Well, consider a point as indicated by the big red arrow here near the “North Pole” of this galaxy.

Gravity’s E should be pulling it downward, toward the center, but according to the only-azimuthal motion-assumption it is not falling down. Yet according to Equation 2.2 above, if the pressure is zero then the only other force left to hold it up is v x B. So of course these assumptions must imply a large magnetic field B, with a comparable influence to E.
But is this right, and if not which of Ludwig’s assumptions is wrong, or at least high questionable? I say it is his assumption of zero pressure, an assumption also made by all of the other related papers I found. Ludwig justifies his zero-pressure assumption by saying that stars almost never collide. But the concept of pressure just doesn’t depend much on collision rates!
Consider that astronomers usually say that what “holds up” stars near the red arrow is momentum. Previously, their velocities started high closer to the center, and declined as they climbed the gravitational potential to reach near that red arrow. They have recently or will soon stop rising and begin to fall back toward the center.
One can tell exactly this same story about atoms in an atmosphere. Even if they never collided, their average density and velocity would still change just the same with altitude as they flew up from the ground. Atmospheric “pressure” declines with altitude because the number of atoms that pass through any given area (and how fast and massive) declines, not because they actually collide. Pressure tells of momentum transfer that would happen if the objects moving through a plane were instead to bounce off that plane; but they don’t actually have to bounce for there to be pressure.
So similarly the usual picture of galaxies “held up” by momentum is actually a picture of a non-zero pressure, a pressure highest near the center and declining away from it, and a pressure strong enough to counter gravity and “hold up” the average density of stars near the North pole. So the pressure is not near zero, even though collisions are very rare.
What about Ludwig’s empirical fits? Well he never compares them to models using non-zero pressure, so his fits don’t tell us which better explains rotation curves, pressure or gravitomagnetism. Same for all the other papers in his area.
So yes, the skeptics were right; Ludwig’s analysis contains a big questionable assumption, and so their quick calculation doesn’t obviously mislead here. And yes if you are busy and this is not your area it makes sense to just ignore his paper if you think it unlikely to be right. But if you are going to publicly denounce it as mistaken, especially on the basis of your high level of physics authority, it is more helpful if you do what I’ve tried to do, namely try to find and publicize its error, or publicly admit if you can’t. That’s how research moves forward.
Added 16Mar: These authors got at least five publications out of their mistakes, and no serious academic journal would consider publishing my rebuttal. As those publications are full of complex math and technical work, and my blog post looks doesn’t show much technical prowess. Which shows a well-known big bias in academia (econ too, not just physics): why bother to learn the concepts deeply if you can get many more publications and prestige via more manipulation of symbols you insufficiently understand? Why bother to look for such errors in others’ work if you can’t get publication credit from it? Even if you do make a conceptual mistake, your referees aren’t likely to notice, and even if someone publicly shows the mistake, that will likely be someone/someplace with too little academic prestige to count, or even be noticed.



Thanks for your commentary.
So it seems Ludwig didn't actually derive the magnitude of the gravitomegnetic effect from GR, he made an (erroneous) assumption from which he inferred that the magnitude must be great enough. Is that correct?
FWIW, pressure is not conventionally about momentum. Say you are sitting on a chair. The chair is under pressure, but it and you are both motionless - and so have no momentum. Pressure is defined in terms of force, not momentum.