Yesterday’s Science has a long paper on an exciting new scaling law. For a century we’ve known that larger organisms have lower metabolisms, and thus lower growth rates. Metabolism goes as size to the power of 3/4 over at least twenty orders of magnitude:
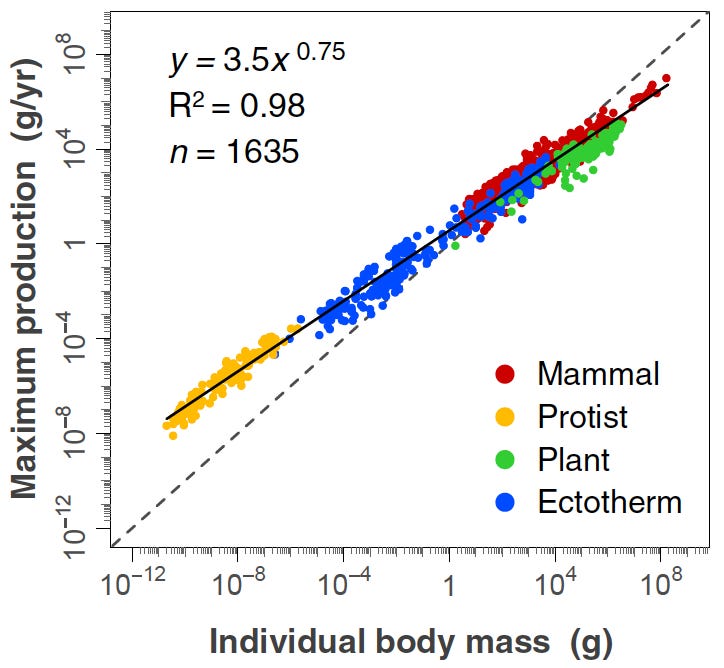
So our largest organisms have a per-mass metabolism one hundred thousand times lower than our smallest organisms.
The new finding is that local metabolism also goes as local biomass density to the power of roughly 3/4, over at least three orders of magnitude. This implies that life in dense areas like jungles is just slower and lazier on average than is life in sparse areas like deserts. And this implies that the ratio of predator to prey biomass is smaller in jungles compared to deserts.
When I researched how to cool large em cities I found that our best cooling techs scale quite nicely, and so very big cities need only pay a small premium for cooling compared to small cities. However, I’d been puzzled about why biological organisms seem to pay much higher premiums to be large. This new paper inspired me to dig into the issue.
What I found is that human engineers have figured ways to scale large fluid distribution systems that biology has just never figured out. For example, the hearts that pump blood through animals are periodic pumps, and such pumps have the problem that the pulses they send through the blood stream can reflect back from joints where blood vessels split into smaller vessels. There are ways to design joints to eliminate this, but those solutions create a total volume of blood vessels that doesn’t scale well. Another problem is that blood vessels taking blood to and from the heart are often near enough to each other to leak heat, which can also create a bad scaling problem.
The net result is that big organisms on Earth are just noticeably sluggish compared to small ones. But big organisms don’t have to be sluggish, that is just an accident of the engineering failures of Earth biology. If there is a planet out there where biology has figured out how to efficiently scale its blood vessels, such as by using continuous pumps, the organisms on that planet will have fewer barriers to growing large and active. Efficiently designed large animals on Earth could easily have metabolisms that are thousands of times faster than in existing animals. So, if you don’t already have enough reasons to be scared of alien monsters, consider that they might have far faster metabolisms, and also be very large.
This seems yet another reason to think that biology will soon be over. Human culture is inventing so many powerful advances that biology never found, innovations that are far easier to integrate into the human economy than into biological designs. Descendants that integrate well into the human economy will just outcompete biology.
I also spend a little time thinking about how one might explain the dependence of metabolism on biomass density. I found I could explain it by assuming that the more biomass there is in some area, the less energy each biomass gets from the sun. Specifically, I assume that the energy collected from the sun by the biomass in some area has a power law dependence on the biomass in that area. If biomass were very efficiently arranged into thin solar collectors then that power would be one. But since we expect some biomass to block the view of other biomass, a problem that gets worse with more biomass, the power is plausibly less than one. Let’s call a this power that relates biomass density B to energy collected per area E. As in E = cBa.
There are two plausible scenarios for converting energy into new biomass. When the main resource need to make new biomass via metabolism is just energy to create molecules that embody more energy in their arrangement, then M = cBa-1, where M is the rate of production of new biomass relative to old biomass. When new biomass doesn’t need much energy, but it does need thermodynamically reversible machinery to rearrange molecules, then M = cB(a-1)/2. These two scenarios reproduce the observed 3/4 power scaling law when a = 3/4 and 1/2 respectively. When making new biomass requires both simple energy and reversible machinery, the required power a is somewhere between 1/2 and 3/4.
Added 14Sep: On reflection and further study, it seems that biologists just do not have a good theory for the observed 3/4 power. In addition, the power deviates substantially from 3/4 within smaller datasets.



Hey. I'm not an expert in physics or anything but I was wondering, do you think the same principles could be used to construct giant trees? I know the height of trees is limited by physics and the way they pump water up to the top. What would plants look like with better pumps?
I never claimed that they were the same category - what I said was that culture is part of biology. Without biology there is no culture. Rocks and stars are examples of things that are not part of biology. Culture is not like that - it is part of living systems.